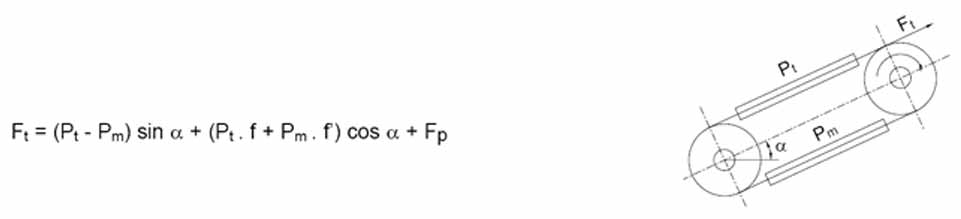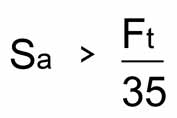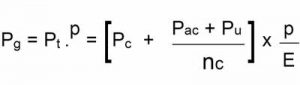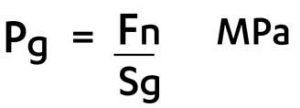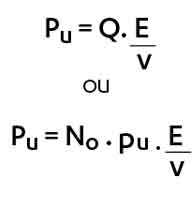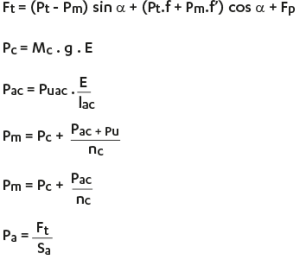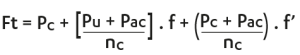► Collect as much data as possible, in particular:
• the chain’s working mode must be perfectly defined, referring to the various possibilities explained above.
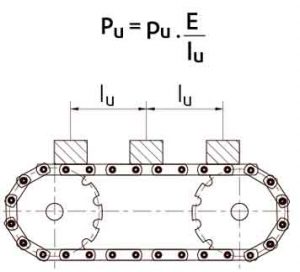
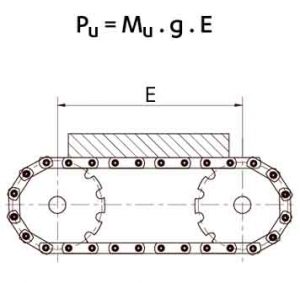
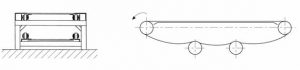
• the weights involved (including that of the chain, which must be estimated from the outset), friction of the chain and of the load being transported, lengths, angles, data concerning any curves, etc.
► Calculate the forces exerted on the chain:
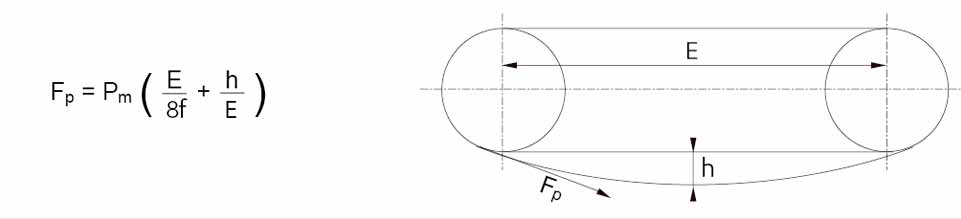
• the traction forces owing to the weights and friction (as well as to any curvature) cause traction constraints in the plates and shearing in the pins, as well as contact pressure between the pins and bushes).
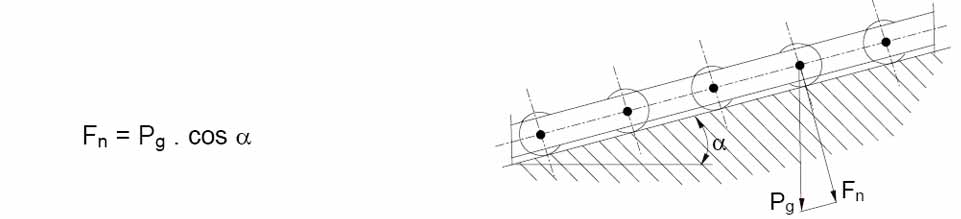
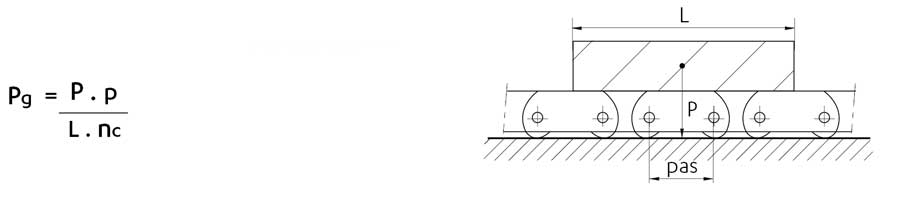
• the normal forces (owing to the weights and any curvature) that cause contact pressure between the rollers and the bushings on the one hand, and the supporting surface on the other hand.
► Choose the chain according to its working mode and the result of the calculations according to one or more of the following criteria:
•the chain’s tensile strength,
• the wear resistance of its hinges and rollers.
► Do the calculations again, introducing the weight of the selected chain if this weight is significantly different from the weight estimated for the first calculations.
► Decide on the technical details for making the installation, referring to the recommendations and making sure that all the working conditions initially stipulated have not changed to such an extent they put the calculations into question.